Mega Millions odds and probabilities for the Mega Millions Jackpot. How to calculate these Mega Millions odds. Jackpot split probabilities including return on investment calculations.

    The mathematical tables on this web page are for the old, pick 5 out of 75, 1 out of 15 game. See the link below for the new pick 5 out of 70, 1 out of 25 game that started Oct. 31, 2017.

http://www.durangobill.com/MegaMillionsOdds.html

   The bottom line is that it is even more difficult to win the new Jackpot. The expected value of the game will still be approximately $0.50 on the dollar - or given the new pricing structure, the new expected return will be about $1.00 returned for each $2.00 that you pay for a ticket.





Ticket Matches       Payout           Odds               Probability
--------------------------------------------------------------------
5 White + Mega      Jackpot    1 in 258,890,850.00      0.000000003863
5 White No Mega   1,000,000    1 in 18,492,203.57       0.00000005408
4 White + Mega        5,000    1 in 739,688.14          0.000001352
4 White No Mega         500    1 in 52,834.87           0.00001893
3 White + Mega           50    1 in 10,720.12           0.00009328
3 White No Mega           5    1 in 765.72              0.001306
2 White + Mega            5    1 in 472.95              0.002114
1 White + Mega            2    1 in 56.47               0.01771
0 White + Mega            1    1 in 21.39               0.04675

Win something      Variable    1 in 14.71               0.0679916



Game Rules
   The numbers picked for the prizes consist of 5 numbers picked at random from a pool of 75 numbers (the White Numbers). Then a single number (the Mega Number) is picked from a second pool that has 15 numbers. If the results of these random number selections match one of the winning combinations on your lottery ticket, then you win something.


Combinatorics Calculations
   In any combinatorics problem where all possible outcomes are equally likely, the probability of a successful outcome is determined by finding the number of successful combinations, and then dividing by the total number of all combinations. There are nine possible configurations that will win something in the Mega Millions Lottery. For each of these, the probability of winning equals the number of winning combinations for that particular configuration divided by the total number of ways the Mega Millions numbers can be picked.


Mega Millions Total Combinations
   Since the total number of combinations for Mega Millions numbers is used in all the calculations, we will calculate it first. The number of ways 5 numbers can be randomly selected from a field of 75 is: COMBIN(75,5) = 17,259,390. (See the math notation page or Help in Microsoft's Excel for more information on “COMBIN”).

   For each of these 17,259,390 combinations there are COMBIN(15,1) = 15 different ways to pick the sixth number (the “Mega” number). The total number of ways to pick the 6 numbers is the product of these. Thus, the total number of equally likely Mega Millions combinations is 17,259,390 x 15 = 258,890,850. We will use this number for each of the following calculations.


Jackpot probability/odds (Payout varies)
   The number of ways the first 5 numbers on your lottery ticket can match the 5 White numbers is COMBIN(5,5) = 1. The number of ways your final number can match the Mega number is: COMBIN(1,1) = 1. The product of these is the number of ways you can win the Jackpot:  COMBIN(5,5) x COMBIN(1,1) = 1. The probability of success is thus: 1/258,890,850 = 0.0000000038626316844. If you express this as “One chance in ???”, you just divide “1” by the 0.0000000038626316844, which yields “One chance in 258,890,850”.

Match all 5 White numbers but not the Mega number (Payout = $1,000,000)
   The number of ways the 5 first numbers on your lottery ticket can match the 5 White numbers is COMBIN(5,5) = 1. The number of ways your final number can match any of the 14 losing Mega numbers is: COMBIN(14,1) = 14. (Pick any of the 14 losers.) Thus there are COMBIN(5,5) x COMBIN(14,1) = 14 possible combinations. The probability for winning $1,000,000 is thus 14/258,890,850 = .00000005407684358 or “One chance in 18,492,203.57”.

Match 4 out of 5 White numbers and match the Mega number (Payout = $5,000)
   The number of ways 4 of the 5 first numbers on your lottery ticket can match the 5 White numbers is COMBIN(5,4) = 5. The number of ways your fifth initial number can match any of the 70 losing White numbers is COMBIN(70,1) = 70.  The number of ways your final number can match the Mega number is: COMBIN(1,1) = 1. The product of these is the number of ways you can get this configuration:  COMBIN(5,4) x COMBIN(70,1) x COMBIN(1,1) = 350. The probability of success is thus: 350/258,890,850  = 0.00000135192109 or “One chance in 739,688.14”.

Match 4 out of 5 White numbers but not match the Mega number (Payout = $500)
   The number of ways 4 of the 5 first numbers on your lottery ticket can match the 5 White numbers is COMBIN(5,4) = 5. The number of ways your fifth initial number can match any of the 70 losing White numbers is COMBIN(70,1) = 70.  The number of ways your final number can match any of the 14 losing Mega numbers is: COMBIN(14,1) = 14. The product of these is the number of ways you can get this configuration:  COMBIN(5,4) x COMBIN(70,1) x COMBIN(14,1) = 4,900. The probability of success is thus: 4,900/258,890,850 = 0.000018926895 or “One chance in 52,834.87”.

Match 3 out of 5 White numbers and match the Mega number (Payout = $50)
   The number of ways 3 of the 5 first numbers on your lottery ticket can match the 5 White numbers is COMBIN(5,3) = 10. The number of ways the 2 losing initial numbers on your ticket can match any of the 70 losing White numbers is COMBIN(70,2) = 2,415.  The number of ways your final number can match the Mega number is: COMBIN(1,1) = 1. The product of these is the number of ways you can get this configuration:  COMBIN(5,3) x COMBIN(70,2) x COMBIN(1,1) = 24,150. The probability of success is thus: 24,150/258,890,850 = 0.000093282555 or One chance in 10,720.12”.

Match 3 out of 5 White numbers but not match the Mega number (Payout = $5)
   The number of ways 3 of the 5 first numbers on your lottery ticket can match the 5 White numbers is COMBIN(5,3) = 10. The number of ways the 2 losing initial numbers on your ticket can match any of the 70 losing White numbers is COMBIN(70,2) = 2,415.  The number of ways your final number can match any of the 14 losing Mega numbers is: COMBIN(14,1) = 14. The product of these is the number of ways you can get this configuration:  COMBIN(5,3) x COMBIN(70,2) x COMBIN(14,1) = 338,100. The probability of success is thus: 338,100/258,890,850 = 0.001306 or “One chance in 765.72”.

Match 2 out of 5 White numbers and match the Mega number (Payout = $5)
   The number of ways 2 of the 5 first numbers on your lottery ticket can match the 5 White numbers is COMBIN(5,2) = 10. The number of ways the 3 losing initial numbers on your ticket can match any of the 70 losing White numbers is COMBIN(70,3) = 54,740.  The number of ways your final number can match the Mega number is: COMBIN(1,1) = 1. The product of these is the number of ways you can get this configuration:  COMBIN(5,2) x COMBIN(70,3) x COMBIN(1,1) = 547,400. The probability of success is thus: 547,400/258,890,850  = 0.0021144 or “One chance in 472.95”.

Match 1 out of 5 White numbers and match the Mega number (Payout = $2)
   The number of ways 1 of the 5 first numbers on your lottery ticket can match the 5 White numbers is COMBIN(5,1) = 5. The number of ways the 4 losing initial numbers on your ticket can match any of the 70 losing White numbers is COMBIN(70,4) = 916,895.  The number of ways your final number can match the Mega number is: COMBIN(1,1) = 1. The product of these is the number of ways you can get this configuration:  COMBIN(5,1) x COMBIN(70,4) x COMBIN(1,1) = 4,584,475. The probability of success is thus: 4,584,475/258,890,850  = 0.017708 or “One chance in 56.47”.

Match 0 out of 5 White numbers and match the Mega number (Payout = $1)
   The number of ways 0 of the 5 first numbers on your lottery ticket can match the 5 White numbers is COMBIN(5,0) = 1. The number of ways the 5 losing initial numbers on your ticket can match any of the 70 losing White numbers is COMBIN(70,5) = 12,103,014.  The number of ways your final number can match the Mega number is: COMBIN(1,1) = 1. The product of these is the number of ways you can get this configuration:  COMBIN(5,0) x COMBIN(70,5) x COMBIN(1,1) = 12,103,014. The probability of success is thus: 12,103,014/258,890,850  = 0.04675 or “One chance in 21.39”.

Probability of winning something
   If we add all the ways you can win something we get:
1 + 14 + 350 + 4,900 + 24,150 + 338,100 + 547,400 + 4,584,475 + 12,103,014 = 17,602,404 different ways of winning something. If we divide by the 258,890,850, we get .0679916 as a probability of winning something.  1 divided by 0.0679916 yields “One chance in 14.71” of winning something.

Corollary
   You can get a close estimate for the number of tickets that were in play for any given game by multiplying the announced number of “winners” by the above 14.71. Thus, if the lottery officials proclaim that a given lottery drawing had 5 million “winners”, then there were about 5,000,000 x 14.71 ~= 73,500,000 tickets purchased that did not win the Jackpot. Alternately, there were about 73,500,000 - 5,000,000 ~= 68,500,000 tickets that did not win anything.

Note: This web page had over 50,000 hits for the large Jackpot on Jan. 4, 2011. If this is representative of what happened at Mega Millions headquarters, I extend my deepest sympathy to their computers.




Note: All calculations assume that the numbers on any given ticket are picked randomly. In practice, many people pick numbers based on family birthdays, etc., and thus many tickets will have a preponderance of low numbers. As a consequence, the probabilities of a single Jackpot winner will be somewhat lower and the probabilities of no winner or multiple winners will tend to be slightly higher than the numbers shown below. Also if the numbers picked in the drawing are clustered at the high end of the 1-75 range, there will tend to be relatively less “partial match” winners. The reverse will hold true if the drawing numbers cluster in the low end of the number range.



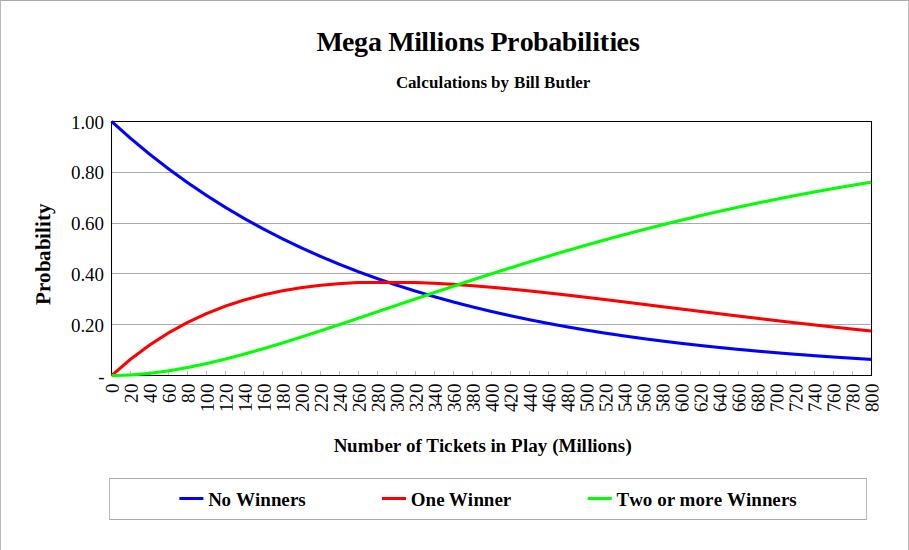
   The above chart shows the probabilities of “No Winners”, “One Winner”, and “Two or more Winners” for various numbers of tickets in play.

   Each entry in the following table shows the probability of “K” tickets holding the same winning Jackpot combination given that "N" tickets are in play for a given Mega Millions game. It is assumed that the number selections on each ticket are picked randomly. For example: If 100,000,000 tickets are in play for a Mega Millions game, then there is a 0.0507 probability that exactly two of these tickets will have the same winning combination.

Note: You can get a rough estimate of the number of tickets in play as follows. If the preceding Mega Millions game had no Jackpot winner, multiply the dollar increase in the advertised Jackpot from the preceding game to the current game by 2. (Use the advertised annuity payout amount.) (Technically, this 2 to 1 multiple is variable. If interest rates increased to 4 1/4 % the ratio would be 1.5 to 1. If interest rates were just under 8 %, the ratio would be near 1 to 1.)

    For example, if the preceding game had an advertised annuity payout amount of $200,000,000 and the current game has an advertised annuity payout amount of $220,000,000, then there are about 2 x (220,000,000 – 200,000,000) = 40,000,000 tickets in play for the current game. (Past Jackpot amounts and ticket sales can be seen at: http://www.lottoreport.com/mmsales.htm. The cash payout value for these amounts would be about one-half the announced Jackpot amount.)

“N”   Number                           “K”
of tickets         Number of tickets holding the Jackpot combination
in play            0       1       2       3       4       5       6
----------------------------------------------------------------------
20,000,000      0.9257  0.0715  0.0028  0.0001  0.0000  0.0000  0.0000
40,000,000      0.8568  0.1324  0.0102  0.0005  0.0000  0.0000  0.0000
60,000,000      0.7931  0.1838  0.0213  0.0016  0.0001  0.0000  0.0000
80,000,000      0.7342  0.2269  0.0351  0.0036  0.0003  0.0000  0.0000
100,000,000     0.6796  0.2625  0.0507  0.0065  0.0006  0.0000  0.0000
120,000,000     0.6291  0.2916  0.0676  0.0104  0.0012  0.0001  0.0000
140,000,000     0.5823  0.3149  0.0851  0.0153  0.0021  0.0002  0.0000
160,000,000     0.5390  0.3331  0.1029  0.0212  0.0033  0.0004  0.0000
180,000,000     0.4989  0.3469  0.1206  0.0279  0.0049  0.0007  0.0001
200,000,000     0.4618  0.3568  0.1378  0.0355  0.0069  0.0011  0.0001
220,000,000     0.4275  0.3633  0.1544  0.0437  0.0093  0.0016  0.0002
240,000,000     0.3957  0.3669  0.1700  0.0525  0.0122  0.0023  0.0003
260,000,000     0.3663  0.3679  0.1847  0.0618  0.0155  0.0031  0.0005
280,000,000     0.3391  0.3667  0.1983  0.0715  0.0193  0.0042  0.0008
300,000,000     0.3139  0.3637  0.2107  0.0814  0.0236  0.0055  0.0011
320,000,000     0.2905  0.3591  0.2219  0.0914  0.0283  0.0070  0.0014
340,000,000     0.2689  0.3532  0.2319  0.1015  0.0333  0.0088  0.0019
360,000,000     0.2489  0.3462  0.2407  0.1116  0.0388  0.0108  0.0025
380,000,000     0.2304  0.3382  0.2482  0.1214  0.0446  0.0131  0.0032
400,000,000     0.2133  0.3296  0.2546  0.1311  0.0506  0.0157  0.0040
420,000,000     0.1974  0.3203  0.2598  0.1405  0.0570  0.0185  0.0050
440,000,000     0.1828  0.3106  0.2640  0.1495  0.0635  0.0216  0.0061
460,000,000     0.1692  0.3006  0.2671  0.1582  0.0703  0.0250  0.0074
480,000,000     0.1566  0.2903  0.2692  0.1663  0.0771  0.0286  0.0088
500,000,000     0.1450  0.2800  0.2703  0.1740  0.0840  0.0325  0.0104


Any entry in the table can be calculated using the following equation:

Prob. = COMBIN(N,K) x (Pwin^K) x (Pnotwin^(N-K))

Where:
N = Number of tickets in play
K = Number of tickets holding the Jackpot combination
Pwin = Probability that a random ticket will win ( = 1 / 258,890,850  =  0.00000000386)
Pnotwin = (1.0 - Pwin)  = 0.99999999614
COMBIN(N,K)  = number of ways to select K items from a group of N items
x   =  multiply terms
^   =  raise to power (e.g.  2^3 = 8 )





   For this example we will assume the cash value of the Jackpot is $300,000,000 and there are 300,000,000 tickets in play for the current game. Probability values are from the “300,000,000” row above.

   The first calculation is: “What is the probability that the jackpot will be won?” This is simply (1.00 – the probability that no one will win) = 1.00 – 0.3139 = 0.6861. Thus the expected payout by the lottery is $300,000,000 times 0.6861 = $205,840,234.

   If there are 300,000,000 tickets in play, then we divide the $205,840,234 by 300,000,000 to get an average jackpot payout per ticket of $0.6861. The other smaller prizes add $0.1742 to this amount to give an "expected before tax, cash value of $0.86.

   These calculations can be used to form a table that shows the expected return per ticket ( = expected value per ticket). For example if the cash value of the jackpot is $300,000,000 and there are 300,000,000 tickets in play, then the ticket’s expected value is $0.86.

   The following table shows the "Expected Before Taxes Value" (includes $0.1742 for the smaller prizes) of a $1.00 ticket.


Nbr. Tickets
In Play       < - - - -   Cash Jackpot Size in Millions   - - - - >
In Millions   100   200   300   400   500   600   700   800   900  1000
-----------------------------------------------------------------------
     100     0.49  0.82  1.14  1.46  1.78  2.10  2.42  2.74  3.06  3.38
     200     0.44  0.71  0.98  1.25  1.52  1.79  2.06  2.33  2.60  2.86
     300     0.40  0.63  0.86  1.09  1.32  1.55  1.78  2.00  2.23  2.46
     400     0.37  0.57  0.76  0.96  1.16  1.35  1.55  1.75  1.94  2.14
     500     0.35  0.52  0.69  0.86  1.03  1.20  1.37  1.54  1.71  1.88
     600     0.32  0.47  0.62  0.78  0.93  1.08  1.23  1.38  1.53  1.68
     700     0.31  0.44  0.57  0.71  0.84  0.97  1.11  1.24  1.37  1.51
     800     0.29  0.41  0.53  0.65  0.77  0.89  1.01  1.13  1.25  1.37
     900     0.28  0.39  0.50  0.60  0.71  0.82  0.93  1.04  1.14  1.25
    1000     0.27  0.37  0.47  0.57  0.66  0.76  0.86  0.96  1.06  1.15

   We can also see what happens to the expected value of a ticket if a buying frenzy should develop at this point. Let’s assume that 300 million more tickets are sold. At $1,00 per ticket, the lottery takes in $300 million.1/2 of this goes into the total prize pot. (1/3 for the jackpot and 1/6 for the smaller prizes.) The jackpot is now worth $300 million plus $100 million = $400 million.

   Thus the game is transformed into 600 million tickets in play for a cash jackpot that is now worth $400 million. If we follow the 600-million row to the right until we reach the $400 million column, we find an expected cash jackpot value of $0.78. The buying frenzy has reduced the expected value of a ticket from $0.86 to $0.75.






Finally, it is interesting to calculate what the long term expected return is for each $1.00 lottery ticket that you buy

   The first task is to construct a table where each row lists the winning combination, the payout, the probability of this payout, and the contribution to the expected return (Equals payout times probability.) The probabilities are the same ones we derived earlier. An $85,000,000 cash payout (decline the annuity) is assumed for the Jackpot. (Could be your portion of a shared Jackpot.)

Combination         Payout     Probability   Contribution
---------------------------------------------------------
5 White + Mega   $85,000,000   3.86263E-09     $0.3283 
5 White No Mega    1,000,000   5.40768E-08      0.0541
4 White + Mega         5,000   1.35192E-06      0.0068
4 White No Mega          500   1.89269E-05      0.0095
3 White + Mega            50   9.32826E-05      0.0047
3 White No Mega            5   0.001305956      0.0065
2 White + Mega             5   0.002114405      0.0106
1 White + Mega             2   0.017708138      0.0354
0 White + Mega             1   0.046749485      0.0467

Total                          0.025069987      0.5026

Total for last 6 rows                           0.1134
(Used for after tax calculation)

Thus, for each $1.00 that you spend for Mega Millions tickets, you can expect to get back about $0.50. Of course you get to pay taxes on any large payout, so your net return is even less.




   While the above calculation represents an average Mega Millions game, we might ask what the expected after tax return on your investment might be if a huge Jackpot exists. The following analysis assumes the annuity value of the Jackpot is $1 Billion (that’s a “B”) and there are 600 million tickets in play. The cash value for any Jackpot is about one-half the annuity value which brings the real value down to $500,000,000. All prizes of $50,000 and above are reduced 40% to allow for federal and state taxes. Don’t forget that a large prize will throw you into a top tax bracket.

   First, we check the expected value of a ticket in the table that we calculated earlier. Follow the 600-million row until you come to the $500 million column. The expected cash value of a ticket is $0.93. This included $0.1742 for the smaller prizes so $0.1742 has to be subtracted back out. This leaves $0.75 for the Jackpot component. However, this has to be reduced by 40% for taxes. This leaves an expected after tax value of the jackpot of $0.4507.

  Next we include the after tax expected value from the two >= $50,000 prizes. This equals 0.0541+ 0.0068 = 0.0608 less 40% for taxes to give us an additional $0.0365.

   Finally, we add in the expected value for the “Total for last 6 rows” This adds another 0.1134 for our expected return. The sum of these three numbers is the expected after tax return for this particular combination. $0.4507 + $0.0365 + $ 0.1134 = $0.6006 expected after tax return for each $1 that you spend per ticket.





   The average return per $1.00 ticket includes the extremely low probability that you might win a large prize – for example $5,000 or more. As a practical matter, it is unlikely that you will ever buy enough tickets (fork out enough money) to ever have much of a chance for any of the large prizes. Thus it is probable that all you will ever get back from your ticket purchases are piddling small amounts.

    The percentages for these small amounts can be calculated. The table below shows the percentage chances for various “piddling returns”.

If you spend $1,000 to buy 1,000 tickets (1 ticket for each of 1,000 Mega Millions games) there is a:
48.97 % chance that you will get back $100 or less
60.93 % chance that you will get back $105 or less
70.86 % chance that you will get back $110 or less
80.52 % chance that you will get back $117 or less
89.95 % chance that you will get back $135 or less
94.95 % chance that you will get back $155 or less
98.00 % chance that you will get back $567 or less
99.01 % chance that you will get back $602 or less
99.49 % chance that you will get back $616 or less
99.80 % chance that you will get back $659 or less

Even if you buy 1,000 tickets, your chance of winning a $5,000 or larger prize is less than 0.2 %.




Some states use a Megaplier feature to increase non-jackpot prizes by 2, 3, 4 or 5 times; it costs an additional $1 per play.

   If your state has a “Megaplier” and if your state follows the probabilities posted on the Mega Million web site, then a calculation can be made for the expected return if you pay an additional $1.00 to participate in the Megaplier play. To find out the expected return, we construct a table to calculate the average expected multiplier.


Multiple     Odds        Probability      Contribution
   2       1 in 7.5        0.133333         0.266667
   3       1 in 3.75       0.266667         0.800000
   4       1 in 5          0.200000         0.800000
   5       1 in 2.5        0.400000         2.000000
Totals                     1.000000         3.866667

Odds are from the Mega Millions web page: http://www.megamillions.com/how-to-play
Probability = 1 divided by the odds
Contribution = “Multiple” times “Probability”

   1.0 has to be subtracted from this 3.866667 because you would win “1 unit” of the sub prizes just from your simple ticket purchase. This leaves a “bonus contribution multiplier” of just 2.866667.

  Thus we have calculated that the average multiplier is 2.866667. We then multiply the average extra expected return for all the sub-prizes (previously calculated) by this 2.86667 to get the expected return if you buy the “Megaplier” option.

(0.0541 + 0.0068 + 0.0095 + 0.0047 + 0.0065 + 0.0106 + 0.0354 + 0.0467) x 2.866667 = $0.50

Thus if you pay another $1.00 to buy the Megaplier option, your expected before tax return is $0.50.



2nd Thoughts
There are about 1.5 automobile caused fatalities for every 100,000,000 vehicle-miles. (2000 to 2005 average data http://en.wikipedia.org/wiki/Transportation_safety_in_the_United_States ) If you drive one mile to the store to buy your Mega Millions ticket and then return home, you have driven two miles. Thus the probability that you will join this statistical group is 2 x 1.5 / 100,000,000 = 0.00000003. This can also be stated as “One in 33,333,333”. Thus, if you drive to the store to buy your Mega Million ticket, your chance of being killed (or killing someone else) is nearly 8 times greater than the chance that you will win the Mega Millions Jackpot.

   Alternately, if you “played” Russian Roulette 100 times per day, every day for 71 years, with Mega Millions Jackpot odds, you would have better than a 99% chance of surviving.


3rd Thoughts
A lottery is a “Zero-sum game”. What one group of participants gains in cash, the other group of participants must lose. If we made a list of all the participants in a lottery, it might include:

1) Federal Government (Lottery winnings are taxable)
2) State Governments (Again lottery winnings are taxable)
3) State Governments (Direct share of lottery ticket sales)
4) Merchants that sell tickets (Paid by the lottery organizers)
5) Lottery companies (Hint: They are not doing all this for free)
6) Advertisers and promoters (Paid by the lottery companies)
7) Lottery ticket buyers (Buy lottery tickets and receive payouts)

The winners in the above list are:
1) Federal Government
2) State Government (Taxes)
3) State Government (Direct share)
4) Merchants that sell tickets
5) Lottery companies
6) Advertisers and promoters

And the losers are:
    (Mathematically challenged and proud of it)

Also please see the related calculations for Powerball.


 

Return to Durango Bill's Home page.


Web page generated via Sea Monkey's Composer HTML editor
within  a Linux Cinnamon Mint 18 operating system.
(Goodbye Microsoft)